Early Folly
The following letter
was created and offered to various Universities to
verify the accuracy
of the supposition.
It didn't take long
to have it proven as inaccurate but I can't locate the proof correspondence
and can't remember
just who the professor was who shot holes in it.
- - - - - - - - - - - - - - -
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
16 January 1951
TO WHOM IT MAY CONCERN:
We the undersigned Rudell 0.
BLANKENSHIP, Clarence A. SLOPER and Clifford L. GOODWIN this date 16 January
1951 have to our knowledge discovered the means of trisecting any angle
with the use of a straight edge and compass only. The solution was arrived
at in the following manner:
1. Bisect any angle with a line.
2. Construct a perpendicular to
the bisecting line (intersecting both legs of the angle).
3. Construct a second perpendicular
to the bisecting line further from
the apex.
4. Construct on the second perpendicular,
a trisected line which is also bisected by the bisecting line.
5. Draw lines from the points
of intersection (of the first perpendicular and the legs of the angle)through
the outer points of the second trisected perpendicular, to a point on the
bisecting line.
6. From this point draw lines
through the points of trisection to project onto the first perpendicular
to the bisecting line, thus trisecting the first perpendicular.
7. Draw a semi-circle whose diameter
is formed by the segment of the first perpendicular to the bisecting line,
between the legs of the angle.
8. Using the radius of this semi-circle,
strike arcs trisecting the circumference of the semi-circle.
9. Draw a line connecting the
point of trisection of the first perpendicular and the corresponding
point of trisection of the semi-circle.
10. Bisect this line with a perpendicular
and extend it to intersect with the first perpendicular to the first bisecting
line.
l1. From this point of intersection,
strike an arc which intersects the two corresponding points of trisection.
12. Using as a radius a distance
from the apex of the angle to the point of intersection of one leg and
the perpendicular to the first bisecting line; strick the arc of the angle
to be trisected.
13. This arc will intersect the
arc which passes through the two corresponding points of trisection, at
the point of trisection of the arc of the angle to be trisected.
14. Using the chord of this trisection
as a radius, strike off the two remaining and equal segments of the arc
of the angle to be trisected.
- - - - - - - - - - - - - - -
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
15. Connect these points of trisection
of the arc with the apex of the angle and the angle is trisected.
/s/ Rudell O. Blankenship
/s/ Clarence A. Sloper
/s Clifford L. Goodwin
RUDELL O. BLANKENSHIP
CLARENCE A. SLOPER
CLIFFORD L. GOODWIN
On January 16, 1951, we have personally
witnessed Rudell O. BLANKENSHIP, Clarence A. SLOPER and Clifford L. GOODWIN
work on, and to our knowledge, successfully completed the trisecting of
angles with the use of the above method.
/s/ Carl E. Bolduc
/s/ R. Vaingat
/s/ John W. Baillie
CARL E.BOLDUC
R. VAINGAT
JOHN W. BAILLIE
STATE OF NEW YORK
COUNTY OF NIAGARA
CITY OF NIAGARA FALLS, N.Y.
Sworn to before me on this 17th
day of January 1951
/s/ Margaret Mary
Garrow
Notary Public
MARGARET MARY GARROW Reg. No.
1767
Notary Public, State of New York
Residing in Niagara County at
time of appointment
Certificate filed in Erie County
My Commission Expires March 30,
1951
Editor's note "What did he say?"
Come on, that was over 50 years
ago!
March 1, 2009 - I just had a comment
from Jim Loy who forwarded the following
URL for his treatment of the
Trisection
problem
Those of you more serious in your
studies should visit this extensive sites.
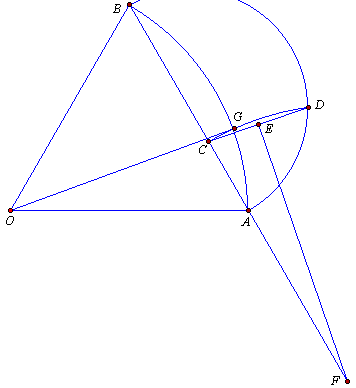
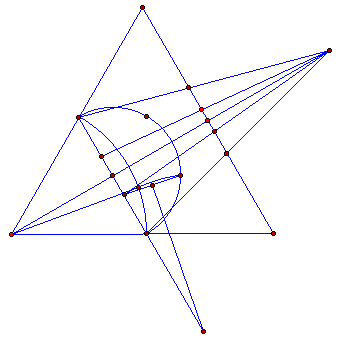
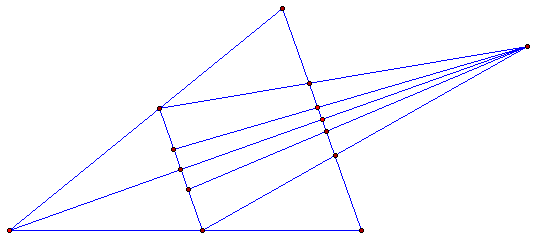
The following three diagrams were
provided by Jim Loy to illustrate the discription of long ago above.
Thanks Jim
- - - - - - - - - - - - - - -
- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -
- - - - - - - - - - - -
Back to
Index Quit


